3.4: Graph Using the y-Intercept and Slope (2024)

Table of Contents

Slope Slope-Intercept Form of a Line Key Takeaways FAQs References

Last updated
Save as PDF

Page ID: 18343

Anonymous
LibreTexts

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}}}$

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}}}$

$ \newcommand{\vectorC}[1]{\textbf{#1}}$

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}}}$

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

Learning Objectives

Identify and find the slope of a line.
Graph a line using the slope and $y$-intercept.

Slope

The steepness of any incline can be measured as the ratio of the vertical change to the horizontal change. For example, a $5$% incline can be written as $\frac{5}{100}$, which means that for every $100$ feet forward, the height increases $5$ feet.

Figure $\PageIndex{1}$

In mathematics, we call the incline of a line the slope and use the letter $m$ to denote it. The vertical change is called the rise and the horizontal change is called the run.

\[\color{Cerulean}{Slope}\quad\color{black}{m=\frac{\text{vertical change}}{\text{horizontal change}}=\frac{rise}{run}}\]

The rise and the run can be positive or negative. A positive rise corresponds to a vertical change up and a negative rise corresponds to a vertical change down. A positive run denotes a horizontal change to the right and a negative run corresponds to a horizontal change to the left. Given the graph, we can calculate the slope by determining the vertical and horizontal changes between any two points.

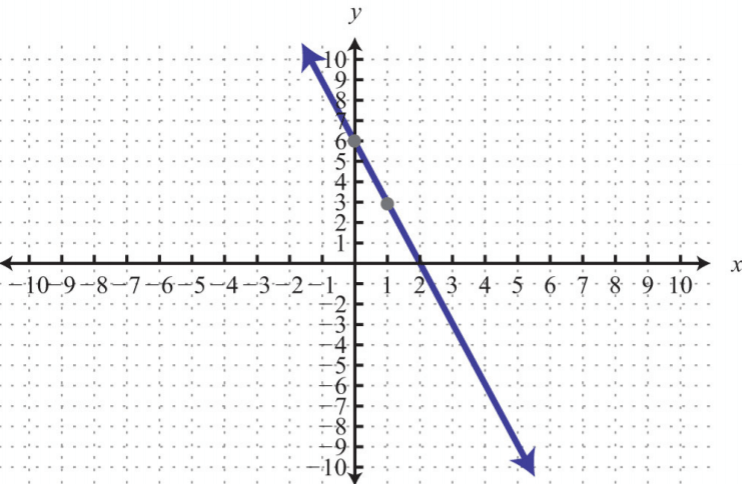
Example $\PageIndex{1}$

Find the slope of the given line:

Figure $\PageIndex{2}$

Solution:

From the given points on the graph, count $3$ units down and $4$ units right.

$m=\frac{rise}{run}=\frac{-3\:\text{units}}{4\:\text{units}}=-\frac{3}{4}$

Answer:

$m=-\frac{3}{4}$

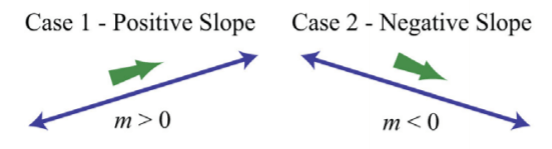
Here we have a negative slope, which means that for every $4$ units of movement to the right, the vertical change is $3$ units downward. There are four geometric cases for the value of the slope.

Figure $\PageIndex{3}$

Reading the graph from left to right, we see that lines with an upward incline have positive slopes and lines with a downward incline have negative slopes.

Figure $\PageIndex{4}$

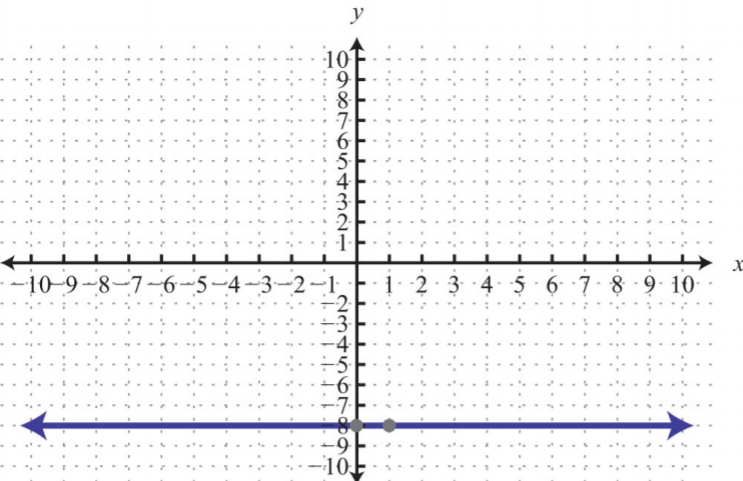
If the line is horizontal, then the rise is $0$:

$m=\frac{rise}{run}=\frac{0}{run}=0$

The slope of a horizontal line is $0$. If the line is vertical, then the run is $0$:

$m=\frac{rise}{run}=\frac{rise}{0}\quad\color{Cerulean}{Undefined}$

The slope of a vertical line is undefined.

Exercise $\PageIndex{1}$

Find the slope of the given line:

Figure $\PageIndex{5}$

Answer: $m=\frac{2}{3}$

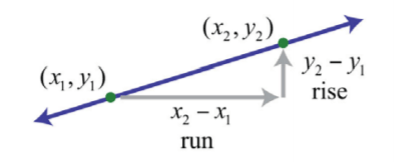
Calculating the slope can be difficult if the graph does not have points with integer coordinates. Therefore, we next develop a formula that allows us to calculate the slope algebraically. Given any two points $(x_{1}, y_{1})$ and $(x_{2}, y_{2})$, we can obtain the rise and run by subtracting the corresponding coordinates.

Figure $\PageIndex{6}$

This leads us to the slope formula. Given any two points $(x_{1}, y_{1})$ and $(x_{2}, y_{2})$, the slope is given by

$m=\frac{rise}{run}=\frac{y_{2}-y_{1}}{x_{2}-x_{1}}$

Example $\PageIndex{2}$

Find the slope of the line passing through $(−3, −5)$ and $(2, 1)$.

Solution:

Given $(−3, −5)$ and $(2, 1)$, calculate the difference of the $y$-values divided by the difference of the $x$-values. Since subtraction is not commutative, take care to be consistent when subtracting the coordinates.

$\begin{array}{cc}{(x_{1},y_{1})}&{(x_{2},y_{2})}\\{(-3,-5)}&{(2,1)}\end{array}$

$\begin{aligned} m&=\frac{y_{2}-y_{1}}{x_{2}-x_{1}} \\ &=\frac{1-(-5)}{2-(-3)} \\ &=\frac{1+5}{2+3} \\ &=\frac{6}{5} \end{aligned}$

Answer:

$m=\frac{6}{5}$

We can graph the line described in the previous example and verify that the slope is $\frac{6}{5}$.

Figure $\PageIndex{7}$

Certainly the graph is optional; the beauty of the slope formula is that we can obtain the slope, given two points, using only algebra.

Slope-Intercept Form of a Line

To this point, we have learned how to graph lines by plotting points and by using the $x$- and $y$-intercepts. In addition, we have seen that we need only two points to graph a line. In this section, we outline a process to easily determine two points using the $y$-intercept and the slope. The equation of any nonvertical line can be written in slope-intercept form $y=mx+b$. In this form, we can identify the slope, $m$, and the $y$-intercept, $(0, b)$.

Example $\PageIndex{7}$

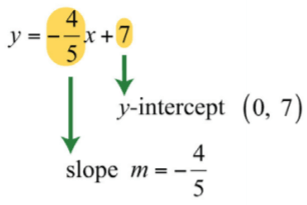
Determine the slope and $y$-intercept:

$y=−\frac{4}{5}x+7$.

Solution:

In this form, the coefficient of $x$ is the slope, and the constant is the $y$-value of the $y$-intercept. Therefore, by inspection, we have

Figure $\PageIndex{9}$

Answer:

The $y$-intercept is $(0, 7)$, and the slope is $m=−\frac{4}{5}$.

It is not always the case that the linear equation is given in slope-intercept form. When it is given in standard form, you have to first solve for $y$ to obtain slope-intercept form.

Example $\PageIndex{8}$

Express $3x+5y=30$ in slope-intercept form and then identify the slope and $y$-intercept.

Solution:

Begin by solving for $y$. To do this, apply the properties of equality to first isolate $5y$ and then divide both sides by $5$.

$\begin{aligned} 3x+5y&=30 \\ 3x+5y\color{Cerulean}{-3x}&=30\color{Cerulean}{-3x} \\ 5y&=-3x+30 \\ \frac{5y}{\color{Cerulean}{5}}&=\frac{-3x+30}{\color{Cerulean}{5}} \\ y&=\frac{-3x}{5}+\frac{30}{5} \\ y&=-\frac{3}{5}x+6 \end{aligned}$

Answer:

Slope-intercept form: $y=−\frac{3}{5}x+6$; $y$-intercept: $(0, 6)$; slope: $m=−\frac{3}{5}$

Once the equation is in slope-intercept form, we immediately have one point to plot, the $y$-intercept. From the intercept, you can mark off the slope to plot another point on the line. From the previous example we have

$y=-\frac{3}{5}x+6$

$y$-intercept: $(0,6)$

slope: $m=-\frac{3}{5}=\frac{-3}{5}=\frac{rise}{run}$

Starting from the point $(0, 6)$, use the slope to mark another point $3$ units down and $5$ units to the right.

Figure $\PageIndex{10}$

It is not necessary to check that the second point, (5, 3), solves the original linear equation. However, we do it here for the sake of completeness.

$\begin{aligned} 3x+5y&=30 \\ 3(\color{OliveGreen}{5}\color{black}{)+5(}\color{OliveGreen}{3}\color{black}{)}&=30 \\ 15+15&=30 \\ 30&=30\quad\color{Cerulean}{\checkmark} \end{aligned}$

Marking off the slope in this fashion produces as many ordered pair solutions as we desire. Notice that if we mark off the slope again, from the point $(5, 3)$, then we obtain the $x$-intercept, $(10, 0)$.

Example $\PageIndex{9}$

Graph:

$−x+2y=4$.

Solution:

In this example, we outline the general steps for graphing a line using slope-intercept form.

Step 1: Solve for $y$ to obtain slope-intercept form.

$\begin{aligned} -x+2y&=4 \\ -x+2y\color{Cerulean}{+x}&=4\color{Cerulean}{+x} \\ 2y&=x+4 \\ \frac{2y}{\color{Cerulean}{2}}&=\frac{x+4}{\color{Cerulean}{2}} \\ y&=\frac{1x}{2}+\frac{4}{2} \\ y&=\frac{1}{2}x+2 \end{aligned}$

Step 2: Identify the $y$-intercept and slope.

$y$-intercept: $(0,2)$

slope: $m=\frac{1}{2}=\frac{rise}{run}$

Step 3: Plot the $y$-intercept and use the slope to find another ordered pair solution. Starting from the $y$-intercept, mark off the slope and identify a second point. In this case, mark a point after a rise of $1$ unit and a run of $2$ units.

Figure $\PageIndex{11}$

Step 4: Draw the line through the two points with a straightedge.

Answer:

Figure $\PageIndex{12}$

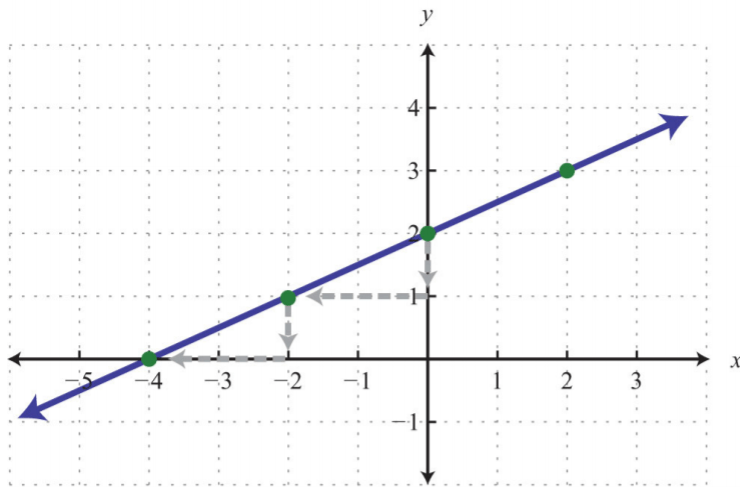
In this example, we notice that we could get the $x$-intercept by marking off the slope in a different but equivalent manner. Consider the slope as the ratio of two negative numbers as follows:

$m=\frac{1}{2}=\frac{-1}{-2}=\frac{rise}{run}$

We could obtain another point on the line by marking off the equivalent slope down $1$ unit and left $2$ units. We do this twice to obtain the $x$-intercept, $(−4, 0)$.

Figure $\PageIndex{13}$

Marking off the slope multiple times is not necessarily always going to give us the $x$-intercept, but when it does, we obtain a valuable point with little effort. In fact, it is a good practice to mark off the slope multiple times; doing so allows you to obtain more points on the line and produce a more accurate graph.

Example $\PageIndex{10}$

Graph and find the $x$-intercept:

$y=\frac{3}{4}x−2$.

Solution:

The equation is given in slope-intercept form. Therefore, by inspection, we have the $y$-intercept and slope.

$y$-intercept: $(0,-2)$

slope: $m=\frac{3}{4}=\frac{rise}{run}$

Figure $\PageIndex{14}$

We can see that the $x$-value of the $x$-intercept is a mixed number between $2$ and $3$. To algebraically find $x$-intercepts, recall that we must set $y = 0$ and solve for $x$.

Answer:

The $x$-intercept is $(2\frac{2}{3}, 0)$.

Example $\PageIndex{11}$

Graph:

$x−y=0$.

Solution:

Begin by solving for $y$.

$\begin{aligned} x-y&=0\\x-y\color{Cerulean}{-x}&=0\color{Cerulean}{-x} \\ -y&=-x \\ \color{Cerulean}{-1\cdot}\color{black}{(-y)}&=\color{Cerulean}{-1\cdot}\color{black}{(-x)} \\ y&=x \end{aligned}$

The equation $y=x$ can be written $y=1x+0$, and we have

$y$-intercept: $(0,0)$

slope: $m=1=\frac{1}{1}=\frac{rise}{run}$

Answer:

Figure $\PageIndex{15}$

Exercise $\PageIndex{3}$

Graph $−2x+5y=20$ and label the $x$-intercept.

Answer

Figure $\PageIndex{16}$

Key Takeaways

Slope measures the steepness of a line as rise over run. A positive rise denotes a vertical change up, and a negative rise denotes a vertical change down. A positive run denotes a horizontal change right, and a negative run denotes a horizontal change left.
Horizontal lines have a slope of zero, and vertical lines have undefined slopes.
Given any two points on a line, we can algebraically calculate the slope using the slope formula, $m=\frac{rise}{run}=\frac{y_{2}−y_{1}}{x_{2}−x_{1}}$.
Any nonvertical line can be written in slope-intercept form, $y=mx+b$, from which we can determine, by inspection, the slope $m$ and $y$-intercept $(0, b)$.
If we know the $y$-intercept and slope of a line, then we can easily graph it. First, plot the $y$-intercept, and from this point use the slope as rise over run to mark another point on the line. Finally, draw a line through these two points with a straightedge and add an arrow on either end to indicate that it extends indefinitely.
We can obtain as many points on the line as we wish by marking off the slope multiple times.

Exercise $\PageIndex{4}$ Slope

Determine the slope and the $y$-intercept of the given graph.

Figure $\PageIndex{17}$

Figure $\PageIndex{18}$

Figure $\PageIndex{19}$

Figure $\PageIndex{20}$

Figure $\PageIndex{21}$

Figure $\PageIndex{22}$

Answer

1. $y$-intercept: $(0, 3)$; slope: $m = −\frac{3}{4}$

3. $y$-intercept: $(0, 2)$; slope: $m = 0$

5. $y$-intercept: $(0, 0)$; slope: $m = 2$

Exercise $\PageIndex{5}$ Slope

Determine the slope, given two points.

$(3, 2)$ and $(5, 1)$
$(7, 8)$ and $(−3, 5)$
$(2, −3)$ and $(−3, 2)$
$(−3, 5)$ and $(7, −5)$
$(−1, −6)$ and $(3, 2)$
$(5, 3)$ and $(4, 12)$
$(−9, 3)$ and $(−6, −5)$
$(−22, 4)$ and $(−8, −12)$
$(\frac{1}{2}, −\frac{1}{3})$ and $(−\frac{1}{2}, \frac{2}{3})$
$(−\frac{3}{4}, \frac{3}{2})$ and $(\frac{1}{4}, −\frac{1}{2})$
$(−\frac{1}{3}, \frac{5}{8})$ and $(\frac{1}{2}, −\frac{3}{4})$
$(−\frac{3}{5}, −\frac{3}{2})$ and $(\frac{1}{10}, \frac{4}{5})$
$(3, −5)$ and $(5, −5)$
$(−3, 1)$ and $(−14, 1)$
$(−2, 3)$ and $(−2, −4)$
$(−4, −4)$ and $(5, 5)$
A roof drops $4$ feet for every $12$ feet forward. Determine the slope of the roof.
A road drops $300$ feet for every $5,280$ feet forward. Determine the slope of the road.
The following graph gives the US population of persons 65 years old and over. At what rate did this population increase from 2000 to 2008?

Figure $\PageIndex{23}$: Source: US Census Bureau.

20. The following graph gives total consumer credit outstanding in the United States. At what rate did consumer credit increase from 2002 to 2008?

Figure $\PageIndex{24}$: Source: US Census Bureau.

21. A commercial van was purchased new for $$20,000$ and is expected to be worth $$4,000$ in 8 years. Determine the rate at which the van depreciates in value.

22. A commercial-grade copy machine was purchased new for $$4,800$ and will be considered worthless in 6 years. Determine the rate at which the copy machine depreciates in value.

23. Find $y$ if the slope of the line passing through $(−2, 3)$ and $(4, y)$ is $12$.

24. Find $y$ if the slope of the line passing through $(5, y)$ and $(6, −1)$ is $10$.

25. Find $y$ if the slope of the line passing through $(5, y)$ and $(−4, 2)$ is $0$.

26. Find $x$ if the slope of the line passing through $(−3, 2)$ and $(x, 5)$ is undefined.

Answer

1. $−\frac{1}{2}$

3. $−1$

5. $2$

7. $−\frac{8}{3}$

9. $−1$

11. $−\frac{33}{20}$

13. $0$

15. Undefined

17. $−\frac{1}{3}$

19. $\frac{1}{2}$ million per year

21. $$2,000$ per year

23. $75$

25. $2$

Exercise $\PageIndex{6}$ Slope-Intercept Form

Express the given linear equation in slope-intercept form and identify the slope and $y$-intercept.

$6x−5y=30$
$−2x+7y=28$
$9x−y=17$
$x−3y=18$
$2x−3y=0$
$−6x+3y=0$
$\frac{2}{3}x−\frac{5}{4}y=10$
$−\frac{4}{3}x+\frac{1}{5}y=−5$

Answer

1. $y=\frac{6}{5}x−6$; slope: $\frac{6}{5}$; $y$-intercept: $(0, −6)$

3. $y=9x−17$; slope: $9$; $y$-intercept: $(0, −17)$

5. $y=\frac{2}{3}x$; slope: $\frac{2}{3}$; $y$-intercept: $(0, 0)$

7. $y=\frac{8}{15}x−8$; slope: $\frac{8}{15}$; $y$-intercept: $(0, −8)$

Exercise $\PageIndex{7}$ Slope-Intercept Form

Graph the line given the slope and the $y$-intercept.

$m=\frac{1}{3}$ and $(0, −2)$
$m=−\frac{2}{3}$ and $(0, 4)$
$m=3$ and $(0, 1)$
$m=−2$ and $(0, −1)$
$m=0$ and $(0, 5)$
$m$ undefined and $(0, 0)$
$m=1$ and $(0, 0)$
$m=−1$ and $(0, 0)$
$m=−\frac{15}{3}$ and $(0, 20)$
$m=−10$ and $(0, −5)$

Answer

Figure $\PageIndex{25}$

Figure $\PageIndex{26}$

Figure $\PageIndex{27}$

Figure $\PageIndex{28}$

Figure $\PageIndex{29}$

Exercise $\PageIndex{8}$ Slope-Intercept Form

Graph using the slope and $y$-intercept.

$y=\frac{2}{3}x−2$
$y=−\frac{1}{3}x+1$
$y=−3x+6$
$y=3x+1$
$y=\frac{3}{5}x$
$y=−\frac{3}{7}x$
$y=−8$
$y=7$
$y=−x+2$
$y=x+1$
$y=\frac{1}{2}x+\frac{3}{2}$
$y=−\frac{3}{4}x+\frac{5}{2}$
$4x+y=7$
$3x−y=5$
$5x−2y=10$
$−2x+3y=18$
$x−y=0$
$x+y=0$
$\frac{1}{2}x−\frac{1}{3}y=1$
$−\frac{2}{3}x+\frac{1}{2}y=2$
$3x+2y=1$
$5x+3y=1$
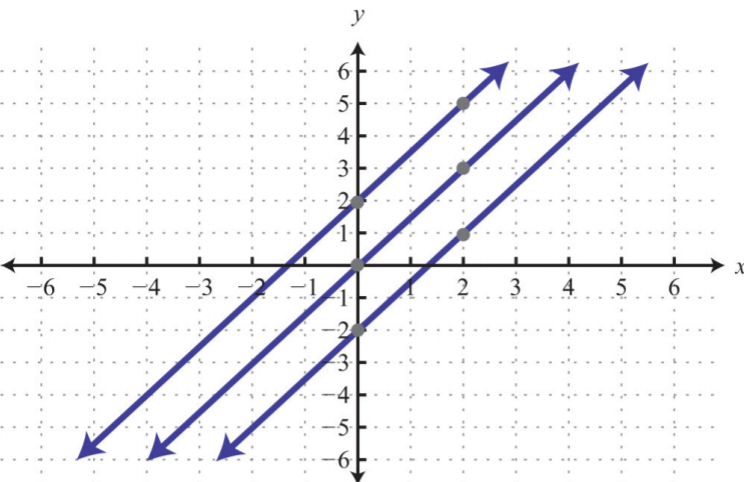
On the same set of axes, graph the three lines, where $y=\frac{3}{2}x+b$ and $b = \{−2, 0, 2\}$.
On the same set of axes, graph the three lines, where $y=mx+1$ and $m = \{−\frac{1}{2}, 0, \frac{1}{2}\}$.

Answer

Figure $\PageIndex{30}$

Figure $\PageIndex{31}$

Figure $\PageIndex{32}$

Figure $\PageIndex{33}$

Figure $\PageIndex{34}$

11.

Figure $\PageIndex{35}$

13.

Figure $\PageIndex{36}$

15.

Figure $\PageIndex{37}$

17.

Figure $\PageIndex{38}$

19.

Figure $\PageIndex{39}$

21.

Figure $\PageIndex{40}$

23.

Figure $\PageIndex{41}$

Exercise $\PageIndex{9}$ Discussion Board Topics

Name three methods for graphing lines. Discuss the pros and cons of each method.
Choose a linear equation and graph it three different ways. Scan the work and share it on the discussion board.
Why do we use the letter m for slope?
How are equivalent fractions useful when working with slopes?
Can we graph a line knowing only its slope?
Research and discuss the alternative notation for slope: $m=\frac{Δy}{Δx}$.
What strategies for graphing lines should be brought to an exam? Explain

Answer

1. Answers may vary

3. Answers may vary

5. Answers may vary

7. Answers may vary

3.4: Graph Using the y-Intercept and Slope (2024)

FAQs

What is the answer to slope y-intercept? ›

In an equation in slope-intercept form (y=mx+b) the slope is m and the y-intercept is b. We can also rewrite certain equations to look more like slope-intercept form. For example, y=x can be rewritten as y=1x+0, so its slope is 1 and its y-intercept is 0.

What is the equation of the line with a slope of 3/4 and y-intercept of 3? ›

Use the slope-intercept formula, y=mx+b, where m is the slope and b is the y intercept. Solution: The equation of the line whose slope is 3/4 and whose y-intercept is-3 in slope-intercept form is y = (3/4)x - 3.

Explore More ›

How do you interpret the slope and the y-intercept of the graph? ›

The slope and y-intercept values indicate characteristics of the relationship between the two variables x and y. The slope indicates the rate of change in y per unit change in x. The y-intercept indicates the y-value when the x-value is 0.

How to calculate a slope? ›

Percent of slope is determined by dividing the amount of elevation change by the amount of horizontal distance covered (sometimes referred to as "the rise divided by the run"), and then multiplying the result by 100.

Show Me More ›

How to solve for y-intercept? ›

Since the y-intercept always has a corresponding x-value of 0, replace x with 0 in the equation and solve for y. On a graph, the y-intercept can be found by finding the value of y when x=0. This is the point at which the graph crosses through the y-axis.

How can the y-intercept and slope of this line be interpreted? ›

The slope and intercept give a lot of information about sets of data. The slope represents the change in y for any 1 unit change in x. The intercept, also known as the y-intercept, is where the line of best fit intersects the y-axis. It represents the initial condition or starting point of the data.

View Details ›

How to find the equation of a line? ›

To find an equation of a line given the slope and a point.

Identify the slope.
Identify the point.
Substitute the values into the point-slope form, y − y 1 = m ( x − x 1 ) . y − y 1 = m ( x − x 1 ) .
Write the equation in slope-intercept form.

May 6, 2020

Read The Full Story ›

How to graph with a slope of? ›

Using Slope to Graph Lines

First, plot the point. Next, if the slope is a fraction, move to the right the number of spaces equal to the denominator, and move up (or down, if the slope is negative) the number of spaces equal to the numerator. Plot a point at the spot you end up.

How do you write an equation in slope-intercept form and graph? ›

Slope intercept form is y=mx+b, where m is slope and b is the y-intercept. We can use this form of a linear equation to draw the graph of that equation on the x-y coordinate plane. Slope intercept form is y = m x + b ‍ , where ‍ is slope and ‍ is the ‍ -intercept.

Discover More ›